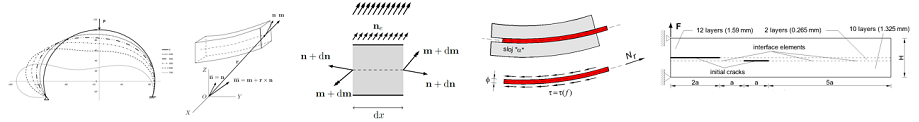
The project is divided into work-packages (WP) in relation to a specific class of problems defined in a particular domain (PD) giving the solution in a particular function space (FS). The outcomes follow from the existing preliminary results obtained by the project group.
WP1. Configuration-dependent interpolation for homogeneous straight and curved 3D beams
- PD: one-dimensional linear (vector) space for straight beams, one-dimensional non-linear space for curved beams
- FS: six-dimensional non-linear manifold involving a three-dimensional vector space of positions, and a three-parametric group of orientations
Team members: Gordan Jelenić, Dragan Ribarić and Edita Papa Dukić
WP2. Configuration-dependent interpolation for homogeneous flat and curved shells
- PD: two-dimensional linear (vector) space for flat shells, two-dimensional non-linear space for curved shells
- FS: five-dimensional non-linear manifold involving a three-dimensional vector space of positions, and a two-dimensional surface of a unit sphere representing rotations of the unit vectors spanning the shell mid-surface around one another
Team members: Gordan Jelenić and Dragan Ribarić
WP3. Configuration-dependent interpolation for straight layered 2D beams including discontinuities
- PD: one-dimensional linear (vector) space
- FS: 3*n-dimensional vector space of two positions and one rotation per layer for each of n layers
Team members: Gordan Jelenić, Leo Škec and Paulo Šćulac
WP4. Configuration-dependent integration of equations of motion
- PD: one-dimensional and two-dimensional linear (vector) and non-linear spaces, three-dimensional vector space in union with a time dimension
- FS: respective function spaces of WP1-WP3, three-dimensional vector space for a 3D continuum
Team members: Gordan Jelenić, Maja Gaćeša and Mohammad Reza Moosavi
WP5. Configuration-dependent interpolation for micro-polar continua (in conjunction with CSF project ‘Young researchers’ career development — Education of new doctors of sciences’)
- PD: three-dimensional linear (vector) space
- FS: six-dimensional non-linear manifold involving a three-dimensional vector space of positions, and a three-parametric group of orientations
Team members: Gordan Jelenić and Sara Grbčić